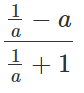Equivalent expression examples |How to find expression equivalent to|

Q.1 Expression equivalent to 2x + 3y?

(a). 2y + 3x

(b).y + y + x + x + x

(c). y + y + y + x + x

(d). None of the above

Answer = (c) = y + y + y + x + x

Explanation:-

Give expression, 2x + 3y

Let’s put the value in given expression for x = 1 and for y = 2.

∴ 2(1) + 3(2) = 8

Now put the same value of x and y in given options

Option (a) = 2(2) + 3(1) = 7

Option (b) = 2 + 2 + 1 + 1 + 1 = 7

Option (c) = 2 + 2 + 2 + 1 + 1 = 8

Hence, the option (c) expression(y + y + y + x + x) equivalent with the given expression(2x + 3y)

Q.2 Expression equivalent to the square root of 80?

(a). 4√5

(b). 5√4

(c). 4√16 × √36

(d). Both (a) and (c)

Answer = (a) = 4√5

Explanation:-

Give expression, √80

Let, x = √80

Square both sides

∴ x² = 80

Now square the each option

option (a) = 4²5 = 80

option (b) = 5²4 = 100

option (c) = 4² × 16 × 36 = 9,216

Hence, the option (a) is the right answer.

Q.3 Expression equivalent to 4b?

(a). b(3 + 1)

(b). b+2(b+2b)

(c). b + b²

(d). None of the above

Answer = (a) = b(3 + 1)

Explanation:-

Give expression, 4b

Let’s put the value in given expression for b = 2

∴ 4(2) = 8

Now put the same value of b in given options

Option (a) = 2(3 + 1) = 8

Option (b) = 2 + 2(2 + 2×2) = 14

Option (c) = 2 + 2² = 6

Hence, option (a) is correct for the given expression.

Q.4 Expression equivalent to (3x⁻²)³?

(a). 3²/x⁴

(b). 3³/x⁴

(c). 27/x⁶

(d). None of the above

Answer = (c) = 27/x⁶

Explanation:-

Give expression, (3x⁻²)³

We have inside the brackets “3 power 1” and “x power -2”

= (3x⁻²)³

A value with a negative exponent is equivalent to its multiplicative inverse

= (3/x²)³

We use exponents laws: all exponents inside the brackets multiply with 3

= 3³/x^{2 × 3}

Finally, we have

= 27/x⁶

Hence, the expression (3x⁻²)³ is equivalent to 27/x⁶

We can also solve this problem by putting the value for x.

Let’s put the value in given expression(3x⁻²)³ for x = 2

∴ (3 × 2^-2)³ = 27/64

Now put the same value of x in given options

Option (a) = 3²/2⁴ = 9/16

Option (b) = 3³/2⁴ = 27/16

Option (c) = 27/2⁶ = 27/64

Hence, the option (c) is correct.

Q.5 Expression equivalent to 4(x + 1)?

(a). 4x + 2

(b). 2(2x + 2)

(c). 2(2x + 1)

(d). None of the above

Answer = (b) = 2(2x + 2)

Explanation:-

Give expression, 4(x + 1)

Let’s put the value in given expression for x = 1.

∴ 4(1 + 1) = 8

Now put the same value of x in given options

Option (a) = 4(1) + 2 = 6

Option (b) = 2(2×1 + 2) = 8

Option (c) = 2(2×1 + 1) = 6

Hence, the option (b) is correct.

Q.6 Expression equivalent to 15x + 25x²?

(a). 5x (3 + 5x)

(b). – 5(3 + 7x)

(c). – 7(5x² + 2x)

(d). None of the above

Answer = (a) = 5x (3 + 5x)

Explanation:-

Give expression, 15x + 25x²

Let’s put the value in given expression for x = 1.

∴ 15(1) + 25(1²) = 40

Now put the same value of x in given options

Option (a) = 5×1(3 + 5×1) = 40

Option (b) = -5(3 + 7×1) = -50

Option (c) = -7(5×1² + 2×1) = -49

Hence, option (a) is equivalent to the given expression.

Q.7 Expression equivalent to 3(-5y + 4)?

(a). 15y – 12

(b). 15 + 12y

(c). -15y + 12

(d). None of the above

Answer = (c) = -15y + 12

Explanation:-

Give expression, 3(-5y + 4)

To solve this, use distributive law.

⇒ A(B + C) = A.B + A.C

∴ 3(-5y + 4) = 3.(-5y) + 3.4

= -15y + 12

Hence, option (C) is correct for the given expression.

Q.8 Expression equivalent to (4 + 7i)(3 + 4i)?

(a). 16 – 37i

(b). -16 + 37i

(c). 12 – 28i

(d). None of the above

Answer = (b) = -16 + 37i

Explanation:-

Give expression, (4 + 7i)(3 + 4i)

As we know, i² = -1

Use the distributive property to solve this expression-

⇒ A(B + C) = A.B + A.C

∴ (4 + 7i)(3 + 4i) = 4*3 + 4*4i + 7i*3 + 7i*4i

= 12 + 16i + 21i + 28i²

= 12 + 37i + 28*(-1)

= 12 – 28 + 37i

= -16 + 37i

Hence, option (b) is a correct equivalent expression for the given expression.

Q.9 Expression equivalent to log18 – log(p + 2)?

(a). log [18/(p + 2)]

(b). log18.log(p+2)

(c). log(18 – p – 2)

(d). None of the above

Answer = (a) = log [18/(p + 2)]

Explanation:-

Give expression, log18 – log(p + 2)

In logarithm if the expression is in the form of log(a/b), then we can write the expression in the form like log(a/b) = loga – logb 
Using the property of logarithmic, we will solve the above expression.
∴ log18 – log(p + 2)

= log [18/(p + 2)]           [∴ loga – logb  = log(a/b)]

Hence, the option(a) is correct for above expression.

Q.10 Expression equivalent to (f + g)(4)?

(a). f(x) + g(4)

(b). f(4) + g(4)

(c). 4(f.g)

(d). None of the above

Answer = (b) = f(4) + g(4)

Explanation:-

Give expression, (f + g)(4)

Use the distributive property to solve this expression-

⇒ A(B + C) = A.B + A.C

∴ (f + g)(4) =  f(4) + g(4)

Hence, the expression (f + g)(4) is equal to f(4) + g(4).

Q.1 Expression equivalent to x/3/(x – 3)?

(a). x/3(x – 9)

(b). x/(3x – 9)

(c). (3x² – 9)

(d). None of the above

Answer = (b). x/(3x – 9)

Explanation:-

Give expression, x/3/(x – 3)

Let’s put the value in given expression for x = 2.

∴ 2/3/(2 – 3) = -2/3

Now put the same value of x in given options

Option (a) = 2/3(2 – 9) = -2/21

Option (b) = 2(3×2 -9) = -2/3

Option (c) = (3×2² – 9) = 3

Hence, option (b) is equivalent to the given complex expression.

Q.2 Expression equivalent to (1÷x⁵/1÷x⁶)⁴?

(a). 4x

(b). x⁴

(c). x^-4

(d). None of the above

Answer = (b) = x⁴

Explanation:-

Give expression, (1÷x⁵/1÷x⁶)⁴

∴ (x)⁴              (∴A÷B/C÷D = A÷B × D÷C)

= x⁴

Hence, option (b) is correct for the given expression.

Q.3 Which expression is equivalent to

(a). 1 – a

(b). -1

(c). +1

(d). 1 + a

Answer = (a) = 1 – a

Explanation:-

Give expression, (1/a – a)/(1/a + 1)

∴ (1 – a^²)/(1 + a)

= (1 – a)(1 + a)/(1+a)            [∴ a² – b² = (1 + a)(1 + b)]

= (1 – a)

Hence, the correct option is (a) for the given expression.