Tabular integration by parts Examples |Where to use integration by parts tabular method|
What is Tabular method for integration by parts?
Integration by parts tabular method is a short method for integration to solve the integral problem quickly, instead of using the lengthy and tedious process of integration by parts traditional method.
The advantage of the tabular integration by parts method is that it can save huge time in solving the problem. It gives the solution fairly accurate than the integration by parts method.
This tabular method is fairly quick to memorize and easy to use. This method is also called the DI method of Integration by parts.
When can I use the Tabular integration by parts method?
The integration by parts tabular method can be applied to any function which is the product of two expressions, where one of the expressions can be differentiated until it gets zero, and another expression can be integrated simultaneously multiple times.
Suppose a function, f(x) = m(x).n(x), from given two expression, one of the expression, let’s take it m(x) should be differentiate multiple times until it reach to zero, and the another expression n(x) should be integrated simultaneously multiple times.
Note: Tabular integration by parts method can also be used where neither of the expression differentiation goes to zero. Example – (∫e2x.sin3x dx) or (∫sin3x.cos4x dx), etc.
Take a look at where we can apply the tabular integration by parts method.
- When Integrand is the product of Polynomial times and something that can be repeatedly integrated. (∫x10.cos x dx)
- Integrand multiple of power function and an exponential function. (∫x².ex dx)
- Integrand multiple of an exponential and trigonometry function. (∫ex.sin x dx)
- Integrand multiple of power function and a trigonometry function. (∫x³.tan x dx)
How to use or apply the Tabular integration by parts method and its formulas?

Let the understand by taking the example of the integral function
\int _ { } ^ { } t ^ { 2 } \sin t dt
Tabular Integration by parts Method
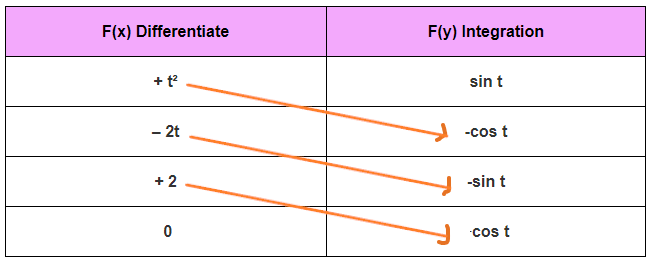
Now we have, F(x) = t2 and F(y) = sint
| F(x) Differentiate | F(y) Integration |
| t² | sin t |
| 2t | -cos t |
| 2 | -sin t |
| 0 | cos t |
Now we will add alternating “+” and “-” signs, starting with “+” on the derivatives side of the table.
| F(x) Differentiate | F(y) Integration |
| + t² | sin t |
| – 2t | -cos t |
| + 2 | -sin t |
| 0 | cos t |
Now Most important Steps in the tabular integration method to find the integral of a given function
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
So, \int _ { } ^ { } t ^ { 2 } \sin t dt = t²(-cost) + (-2t)(-sint) + 2cost + C
= -t²cost + 2tsint + 2cost + C Answer
Tabular Integration by parts Method for solving Definite Integral with limits problems
The definite integral is those integrals that have upper and lower limits or bounds or boundaries. In short, a definite integral has start and end values.
Now, How to solve definite integral problems by the tabular method of integration by parts rather than the traditional method and save our time.
Example 1:
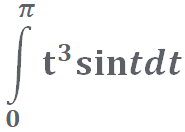
| F(x) Derivative Function | F(y) Integration Function |
| (+) t³ | sin t |
| (-) 3t² | -cos t |
| (+) 6t | -sin t |
| (-) 6 | cos t |
| 0 | sin t |
Follow this procedure:
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them

\int _ { 0 } ^ { \pi } t ^ { 3 } \sin t dt = t³( -cost ) \Big| _ 0 ^ \pi – 3t² ( -sint ) \Big| _ 0 ^ \pi + 6tcost \Big| _ 0 ^ \pi – 6sint \Big| _ 0 ^ \pi
= -t³cost \Big| _ 0 ^ \pi + 3t²sint \Big| _ 0 ^ \pi + 6tcost \Big| _ 0 ^ \pi – 6(sint) \Big| _ 0 ^ \pi
= [-π³cosπ – 0] + [3π².0 – 0] + [6πcosπ – o] + [-6(0 – 0]
= -π³(-1) + 6π(-1) + 0
= π³ – 6π Answer
So, Definite integral of the function \int _ { 0 } ^ { \pi } t ^ { 3 } \sin t dt by tabular integration by parts method is π³ – 6π
Example 2:
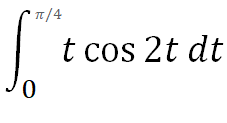
Solution: Using same procedure, Make F(x) = t and F(y) = Cos2t
Now construct the table and take appropriate action to solve these definite integral problems by the tabular method
| F(x) Derivative Function | F(y) Integration Function |
| (+) t | Cos2t |
| (-) 1 | (1/2)Sin2t |
| (+) 0 | -(1/4)Cos2t |
Follow these steps for further solving definite integral by the tabular method
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
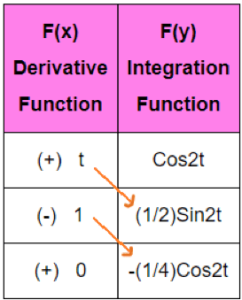
\int _ { 0 } ^ { \frac { \pi } { 4 } } t \cos2t dt = t/2Sin2t \Big| _ 0 ^ \frac { \pi } { 4 } + 1/4Cos2t \Big| _ 0 ^ \frac { \pi } { 4 }
= [ \frac { \pi } { 8 } \sin \frac { \pi } { 2 } – 0 ] + \frac { 1 } { 4 } [ \cos \frac { \pi } { 2 } – \cos0 ]
= \frac { \pi } { 8 } + \frac { 1 } { 4 } ( 0 – 1 )
= \frac { \pi – 2 } { 8 } Answer
Tabular Integration by parts Examples with their Solutions
Let’s take a few more examples to understand the tabular method of integration by parts completely.
Example 1:
Solving problems based on exponential and trigonometric functions using integration by parts tabular method.
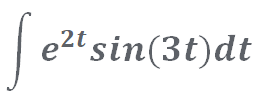
Solution: Now you are thinking that there is no function in the above example that comes up 0 after derivating it endlessly. Then how can we use the tabular integration by parts method here?
Don’t worry just follow the good approach.
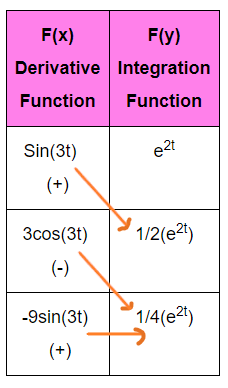
Assume, F(x) = sin3t and F(y) = e2t
Construct tabular method table for integrating this problem
| F(x) Derivative Function | F(y) Integration Function |
| Sin(3t) (+) | e2t |
| 3cos(3t) (-) | 1/2(e2t) |
| -9sin(3t) (+) | 1/4(e2t) |
Now Follow these steps:
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y).
- Multiply the second derivative of F(x) with the second integration of F(y).
- Add all of them
So, \int _ { } ^ { } e ^ { 2t } \sin3t dt = \frac { 1 } { 2 } e2t sin3t – \frac { 3 } { 4 } e2t cos3t – \frac { 9 } { 4 } \int _ { } ^ { } e ^ { 2t } \sin3t dt
⇒ \frac { 13 } { 4 } \int _ { } ^ { } e ^ { 2t } \sin3t dt = \frac { 1 } { 2 } e2t sin3t – \frac { 3 } { 4 } e2t cos3t + C
⇒ \int _ { } ^ { } e ^ { 2t } \sin3t dt = \frac { 1 } { 13 } e2t [ 2sin3t – 3cos3t ] + C Answer
Example 2:
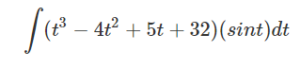
Solution: let’s give a shot at this integral problem by using the integration by parts tabular method.
Look at the problem and choose a function that will give 0 value after derivating multiple times.
F(x) = t3 – 4t2 + 5t + 32 and F(y) = sint
Construct the table to solve this problem with Tabular integration by parts method
| F(x) Derivative function | F(y) Integration function |
| (+) t3 -4t2 + 5t + 32 | sint |
| (-) 3t2 – 8t + 5 | -cost |
| (+) 6t – 8 | -sint |
| (-) 6 | cost |
| 0 | sint |
Use same steps
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
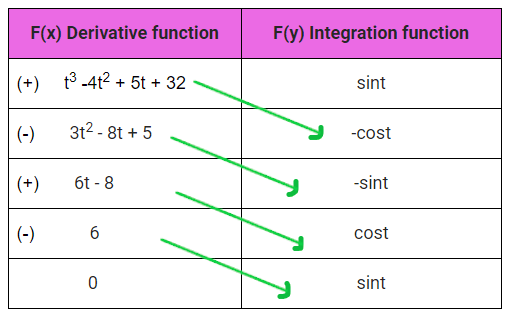
So, we have \int _ { } ^ { } (t ^ { 3 } – 4t ^ { 2 } + 5t + 32)(sint) dt = -(t3 – 4t2 + 5t + 32)(cost) + (3t2 – 8t + 5)(sint) + (6t – 8)(cost) – 6sint + C
Simplify form = (3t2 − 8t − 1)sin(t) + (−t3 + 4t2 + t−40)cos(t) + C Answer
Example 3:
Solving problems based on power and exponential function using integration by parts tabular method.
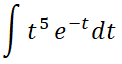
Solution: F(x) = t5 and F(y) = e-t
Construct the table to solve this integral problem with tabular integration by parts method.
| F(x) Derivative Function | F(y) Integration Function |
| (+) t5 | e-t |
| (-) 5t4 | -e-t |
| (+) 20t³ | e-t |
| (-) 60t² | -e-t |
| (+) 120t | e-t |
| (-) 120 | -e-t |
| (+) 0 | e-t |
Use same steps
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
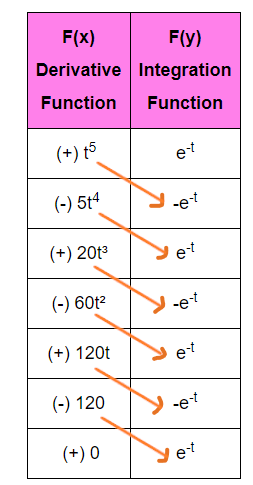
\int _ { } ^ { } t ^ { 5 } e ^ { – t } dt = t5(-e-t) – 5t4(e-t) + 20t3(-e-t) – 60t2(e-t) + 120t(-e-t) – 120(e-t) + C
\int _ { } ^ { } t ^ { 5 } e ^ { – t } dt = -t5(e-t) – 5t4(e-t) – 20t3(e-t) – 60t2(e-t) – 120t(e-t) – 120(e-t) + C
= −(t5 + 5t4 + 20t3 + 60t2 + 120t + 120)e−t + C Answer
Example 4:
Solving problem based on two trigonometric function using Tabular integration by parts method
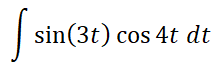
Solution: We know, in the above problem, both function sin3t and cos4t will not get zero regardless of how much time you can differentiate them.
So, take, any of the functions as F(x) and another one as F(y) and apply the Tabular integration method.
So, I assume, F(x) = Sin(3t) and F(y) = Cos(4t) [∴You can choose whatever you want]
Now construct the table for solving this by tabular method
| F(x) Derivative Function | F(y) Integration Function |
| (+) Sin(3t) | Cos(4t) |
| (-) 3Cos(3t) | \frac { 1 } { 4 } Sin(4t) |
| (+) -9Sin(3t) | – \frac { 1 } { 16 } Cos(4t) |
Use these steps solve this integral problem
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y).
- Multiply the second derivative of F(x) with the second integration of F(y).
- Add all of them
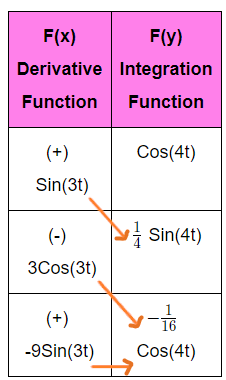
⇒ \int _ { } ^ { } \sin3t \cos4t dt = \frac { 1 } { 4 } Sin(3t)Sin(4t) + \frac { 3 } { 16 } Cos(3t)Cos(4t) + \frac { 9 } { 16 } \int _ { } ^ { } \sin3t \cos4t dt
⇒ \frac { 7 } { 16 } \int _ { } ^ { } \sin3t \cos4t dt = \frac { 1 } { 4 } Sin(3t)Sin(4t) + \frac { 3 } { 16 } Cos(3t)Cos(4t) + C
⇒ \int _ { } ^ { } \sin3t \cos4tdt = \frac { 16 } { 7 } [ \frac { 1 } { 4 } Sin(3t)Sin(4t) + \frac { 3 } { 16 } Cos(3t)Cos(4t) ] + C
= [ \frac { 4 } { 7 } Sin(3t)Sin(4t) + \frac { 3 } { 7 } Cos(3t)Cos(4t) ] + C Answer
Example 5:
Based on when you have only one function in the problem
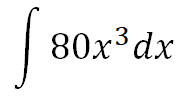
Solution: We can solve this problem by assuming as other function 1 and then apply tabular integration method.
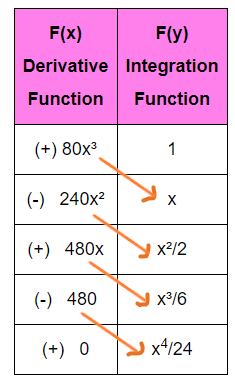
So, let F(x) = 80x³ and F(y) = 1
Construct the table to solve this problem by tabular integration by parts method
| F(x) Derivative Function | F(y) Integration Function |
| (+) 80x³ | 1 |
| (-) 240x² | x |
| (+) 480x | x²/2 |
| (-) 480 | x³/6 |
| (+) 0 | x4/24 |
Use these steps to further solve this integral problem.
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
So, \int _ { } ^ { } 80x ^ { 3 } dx = 80x ^ { 4 } – 240 \frac { x ^ { 4 } } { 2 } + 480 \frac { x ^ { 4 } } { 6 } – 480 \frac { x ^ { 4 } } { 24 }
= 80x ^ { 4 } – 120x ^ { 4 } + 80x ^ { 4 } – 20x ^ { 4 }
= 20x ^ { 4 } + C Answer
Example 6:
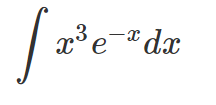
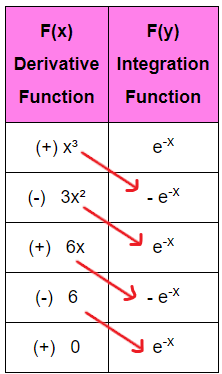
Solution: Let F(x) = x³ and F(y) = e-x
Construct the table to solve this problem using integration by parts tabular method
| F(x) Derivative Function | F(y) Integration Function |
| (+) x³ | e-x |
| (-) 3x² | – e-x |
| (+) 6x | e-x |
| (-) 6 | – e-x |
| (+) 0 | e-x |
Use these steps to further solve this integral problem.
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
\int _ { } ^ { } x ^ { 3 } e ^ { – x } dt = +x3(-e-x) – 3x2(e-x) + 6x(-e-x) – 6(e-x) + C
= – x3.e-x – 3x2.e-x – 6x.e-x – 6.e-x + C Answer
Example 7:
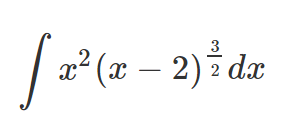
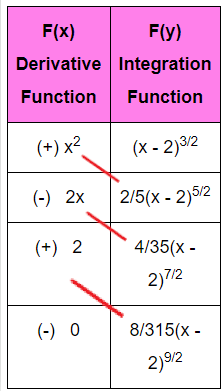
Solution: Let F(x) = x2 and F(y) = (x – 2)3/2
Construct the table to solve this problem by tabular integration by parts method
| F(x) Derivative Function | F(y) Integration Function |
| (+) x2 | (x – 2)3/2 |
| (-) 2x | 2/5(x – 2)5/2 |
| (+) 2 | 4/35(x – 2)7/2 |
| (-) 0 | 8/315(x – 2)9/2 |
Use these steps to further solve this integral problem.
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
\int_{}^{} x^{2}(x – 2)^{\frac{3}{2}}dx = \frac{2}{5}x^{2}(x-2)^{\frac{5}{2}} – \frac{8}{35}x(x-2)^{\frac{7}{2}}+ \frac{16}{315}(x-2)^{\frac{9}{2}} + C Answer
Example 8:

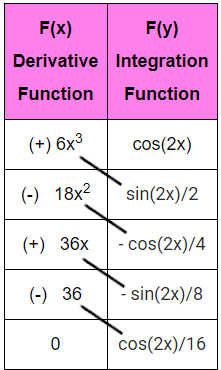
Solution: Let F(x) = 6x3 and F(y) = cos(2x)
Construct the table to solve this problem by tabular integration by parts
| F(x) Derivative Function | F(y) Integration Function |
| (+) 6x3 | cos(2x) |
| (-) 18x2 | sin(2x)/2 |
| (+) 36x | -cos(2x)/4 |
| (-) 36 | -sin(2x)/8 |
| 0 | cos(2x)/16 |
Use these steps to further solve this integral problem.
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
\int_{}^{} 6x^{3}.cos(2x)dx = 3x3sin2x + (9/2)x2cos2x – (9/2)xsin2x – (9/4)cos2x + C Answer
Example 9:
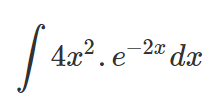
Solution: Let F(x) = 4x2 and F(y) = e-2x
Construct the table to solve this problem by tabular method of integration by parts
| F(x) Derivative Function | F(y) Integration Function |
| (+) 4x2 | e-2x |
| (-) 8x | -e-2x/2 |
| (+) 8 | e-2x/4 |
| (-) 0 | -e-2x/8 |
Use these steps to further solve this integral problem.
- Multiply F(x) with the first integration of F(y)
- Multiply the first derivative of F(x) with the second integration of F(y)………and so on.
- Add all of them
Also check –
- Integration by parts for definite integral
- Integral quotient rule
- Equation of semicircle
- Equivalent expression examples
FAQ
What is the Tabular Integration by parts?The integration by parts tabular method is also called the DI method to solve integration problems quickly by forming three columns, the first one for “Alternative sign”, the second column for “Derivative function” and the third column for “Integration function”. |
Integration by parts vs Tabular integration Method?Integration by parts is the traditional method that is used to find the integral of a product of functions and Tabular integration by parts is a short technique to solve integral problems quickly by letting one of the functions can be differentiated multiple times and the other function can be integrated multiple times with ease. |
When can you use the tabular integration by parts?Tabular integration by parts method can be used where one function is differentiated until it gets zero, and another function can be integrated simultaneously multiple times. Examples – ∫x6.sinx dx, ∫x3.e4x dx, etc. Tabular method of integration by parts can also be used where none of the functions gets zero when differentiated multiple times. Examples – ∫cosx.sinx dx, ∫ex.sin5x dx, etc. |
About the author
Vishal Goyal is the founder of Topblogtenz, a comprehensive resource for students seeking guidance and support in their chemistry studies. He holds a degree in B.Tech (Chemical Engineering) and has four years of experience as a chemistry tutor. The team at Topblogtenz includes experts like experienced researchers, professors, and educators, with the goal of making complex subjects like chemistry accessible and understandable for all. A passion for sharing knowledge and a love for chemistry and science drives the team behind the website. Let's connect through LinkedIn: https://www.linkedin.com/in/vishal-goyal-2926a122b/